Determination of groundwater solute transport parameters in finite element modelling using tracer injection and withdrawal testing data
-
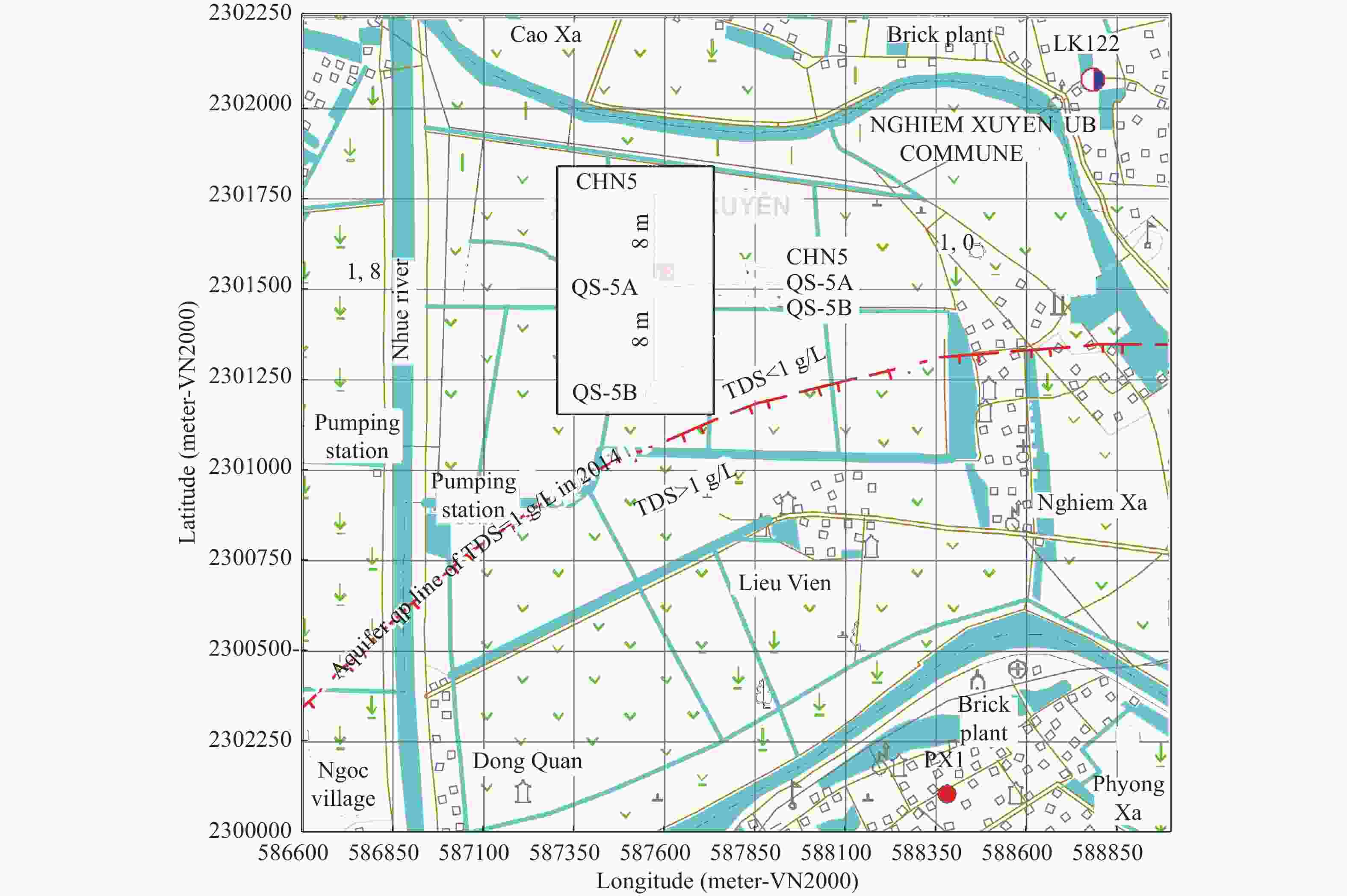
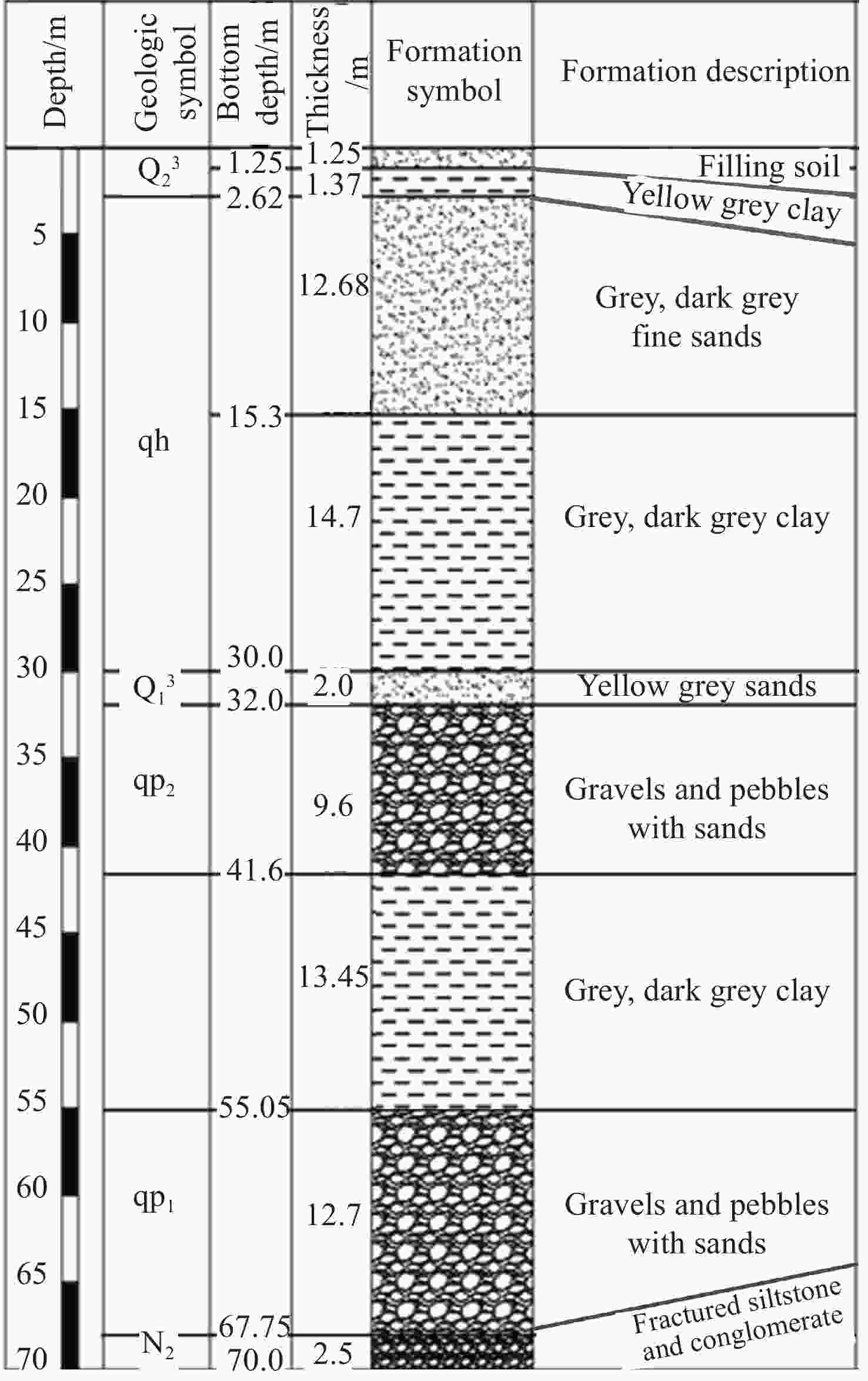
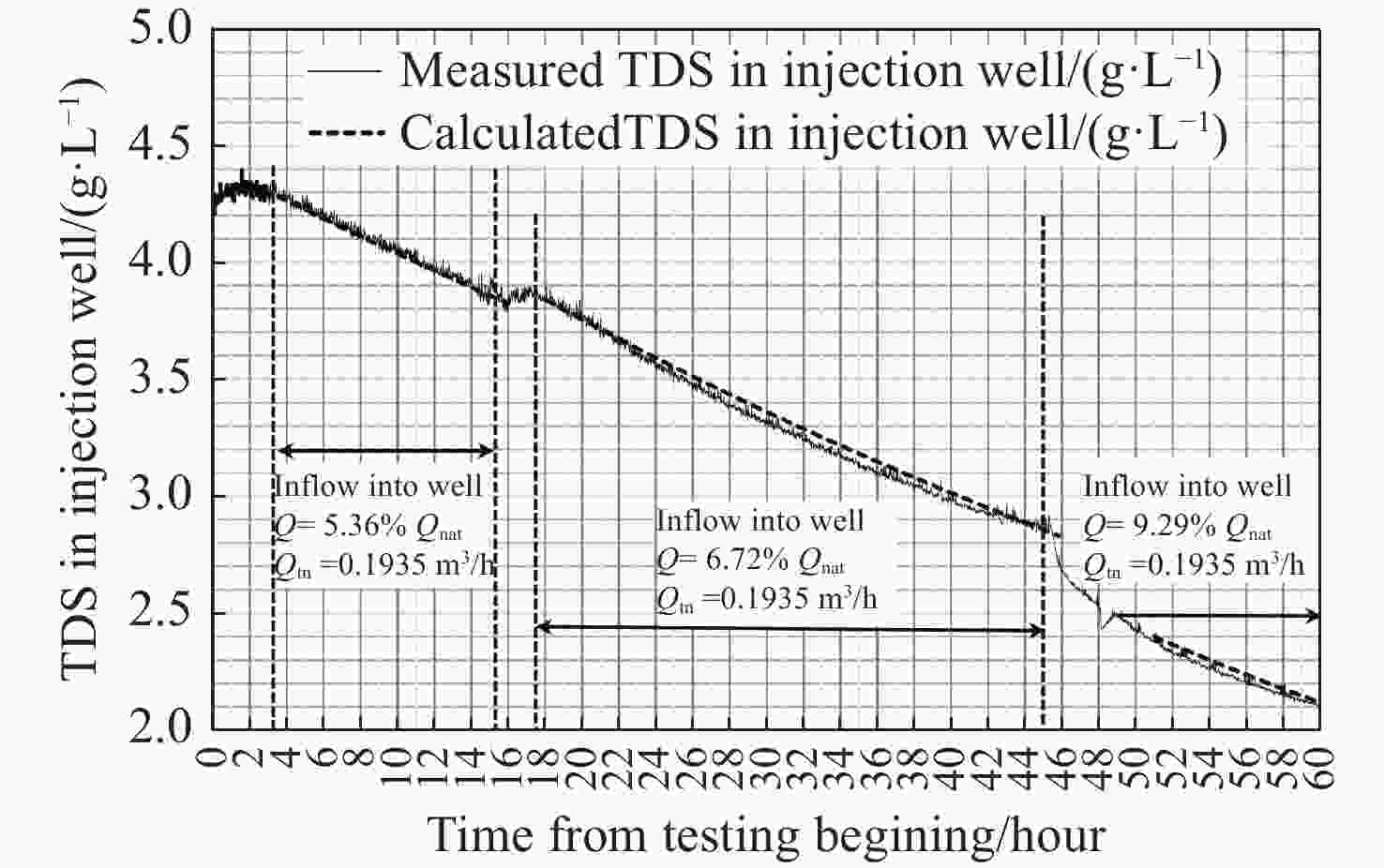
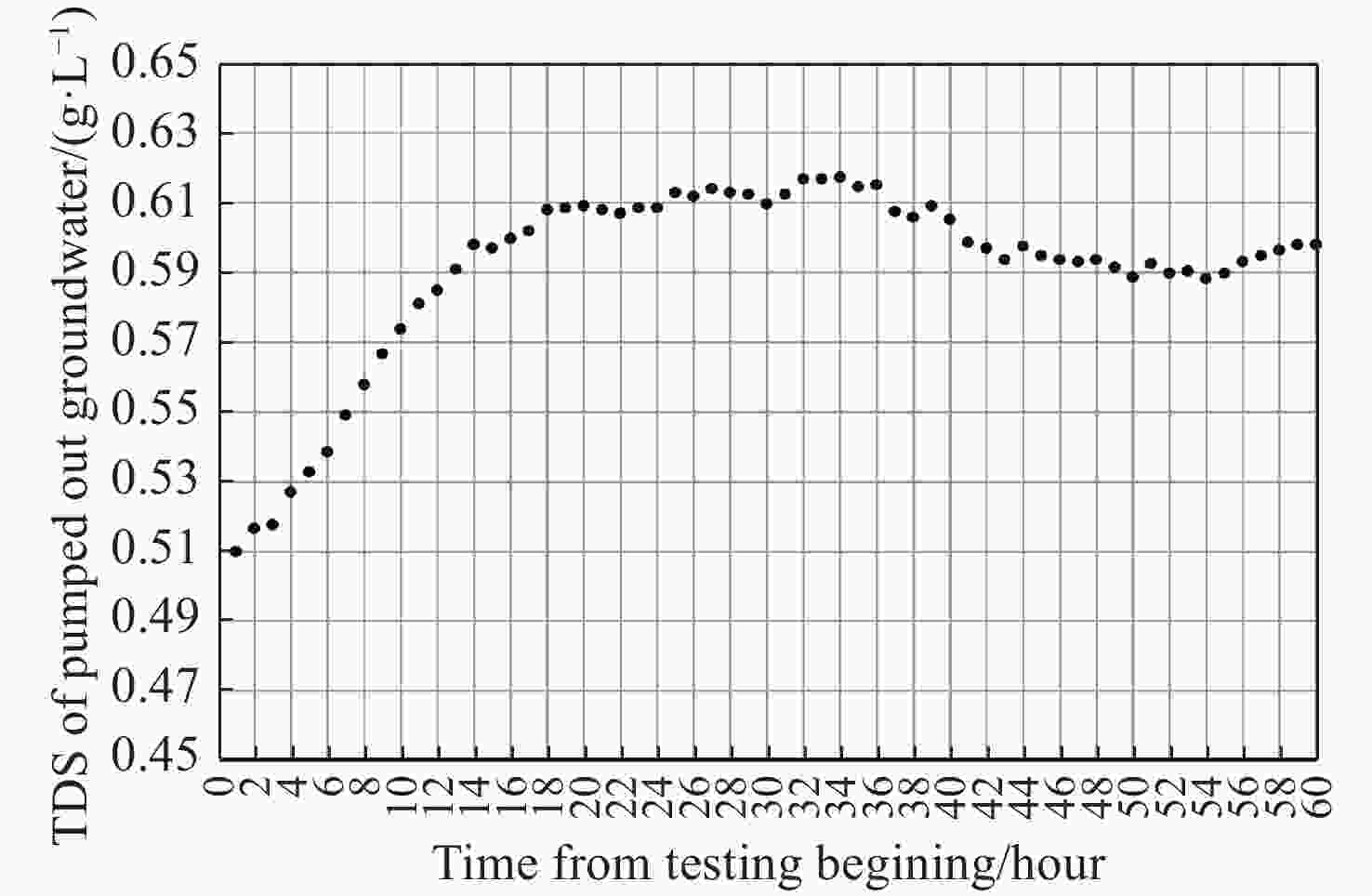
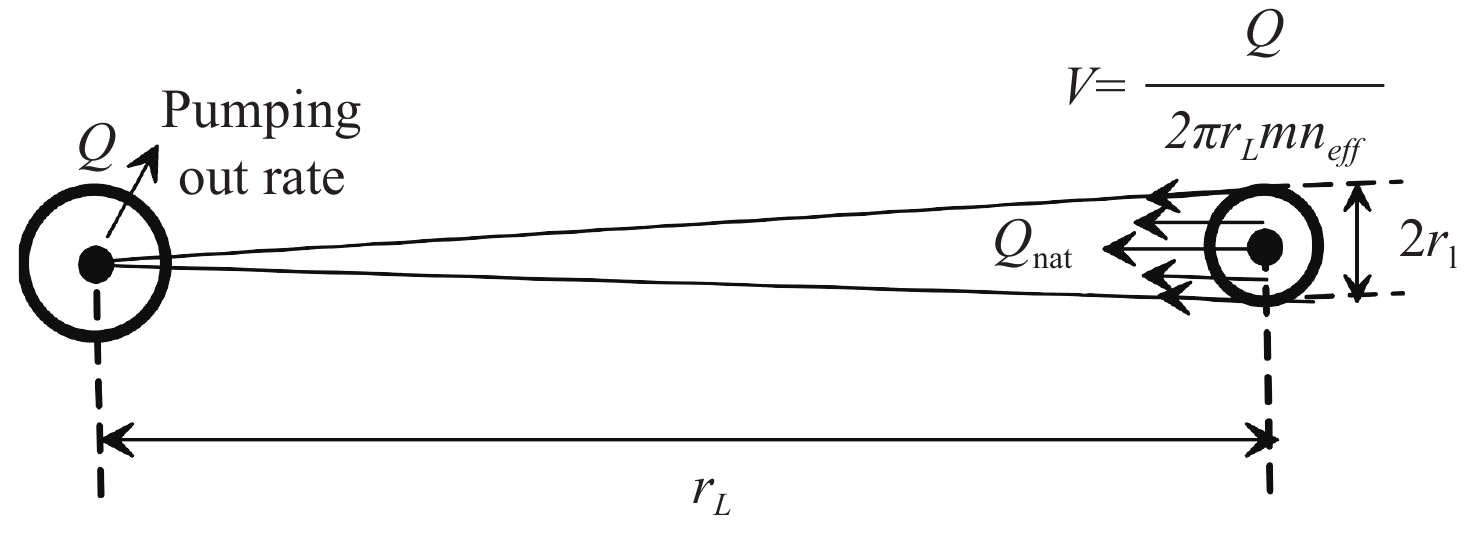
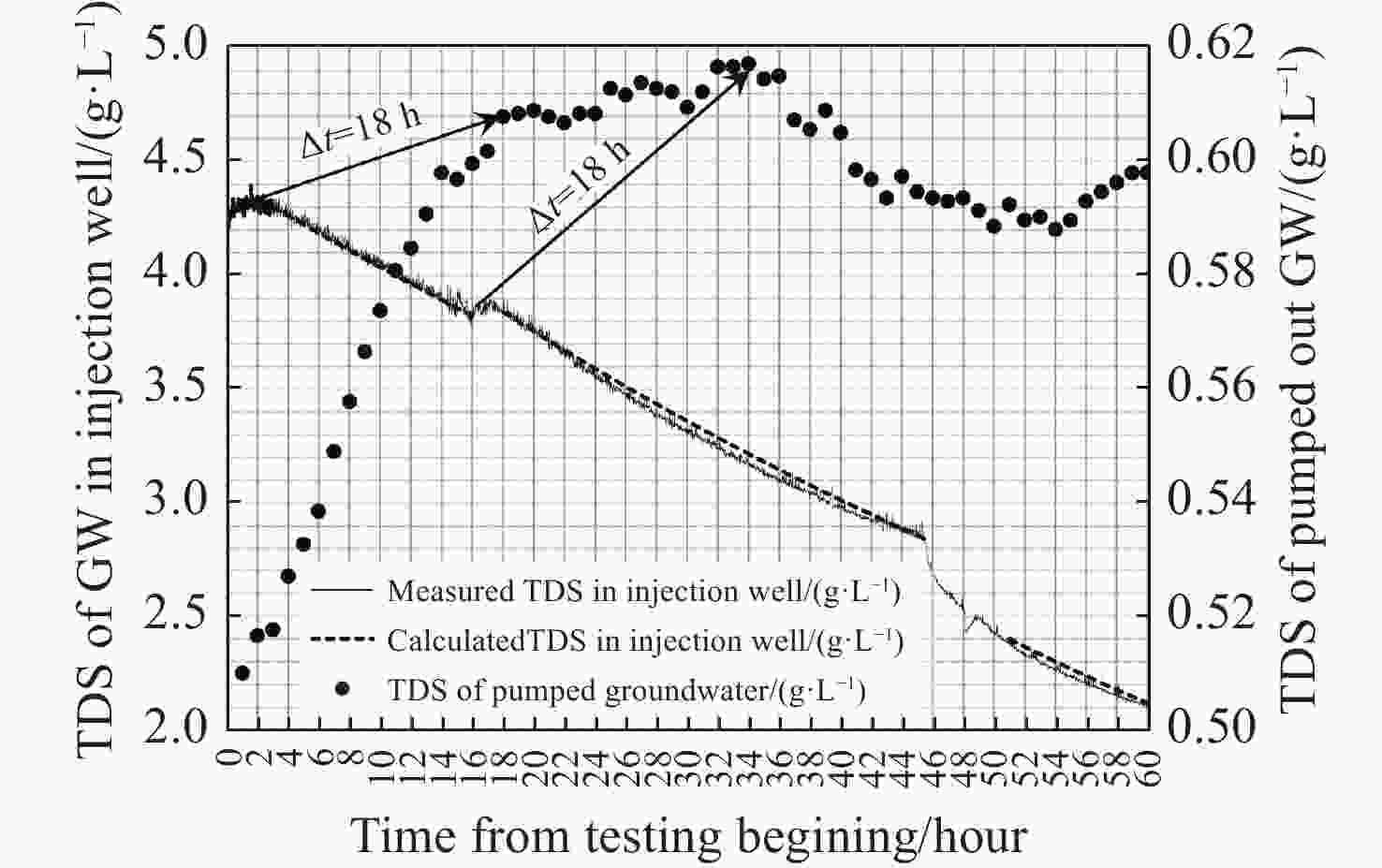
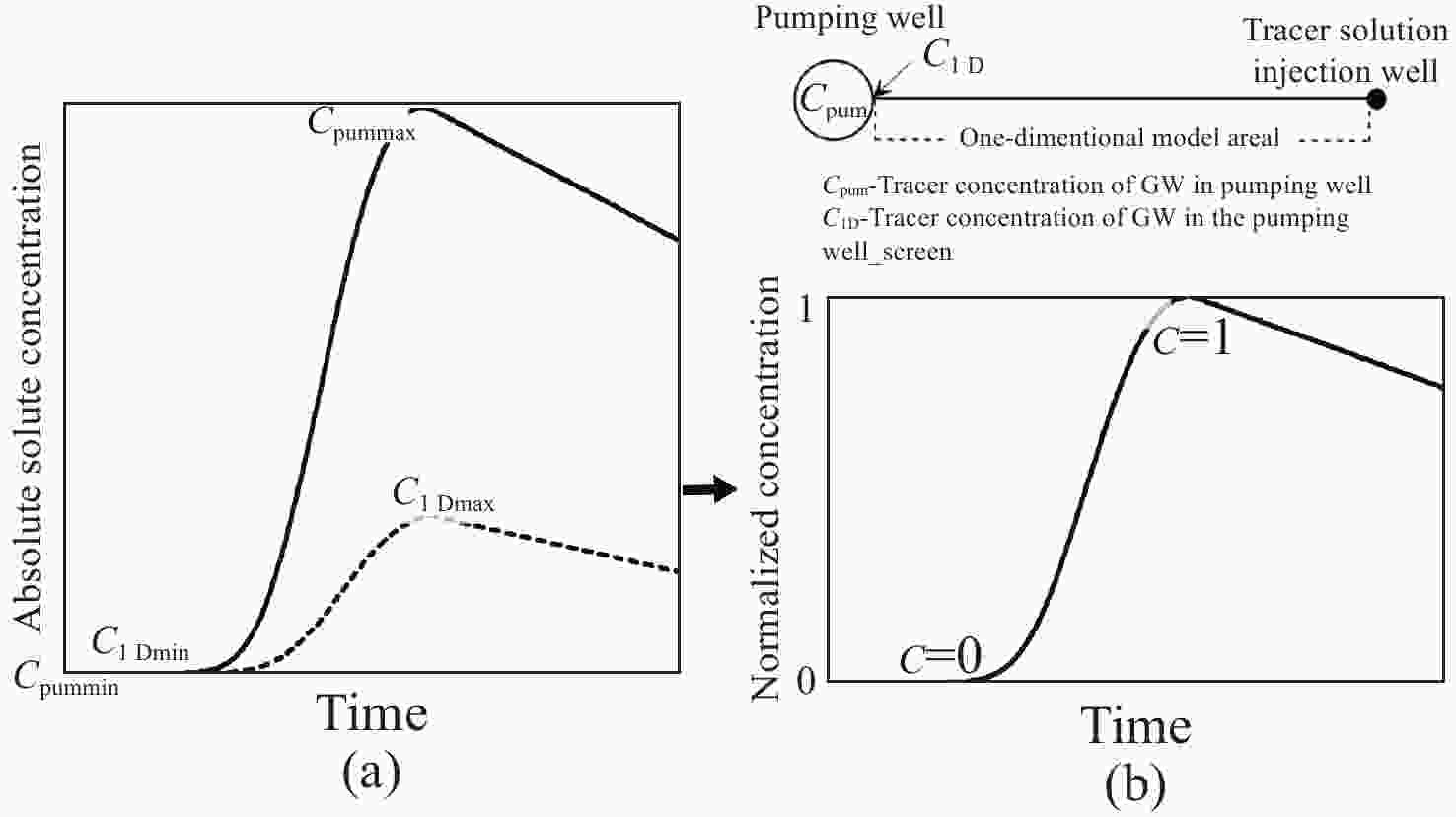
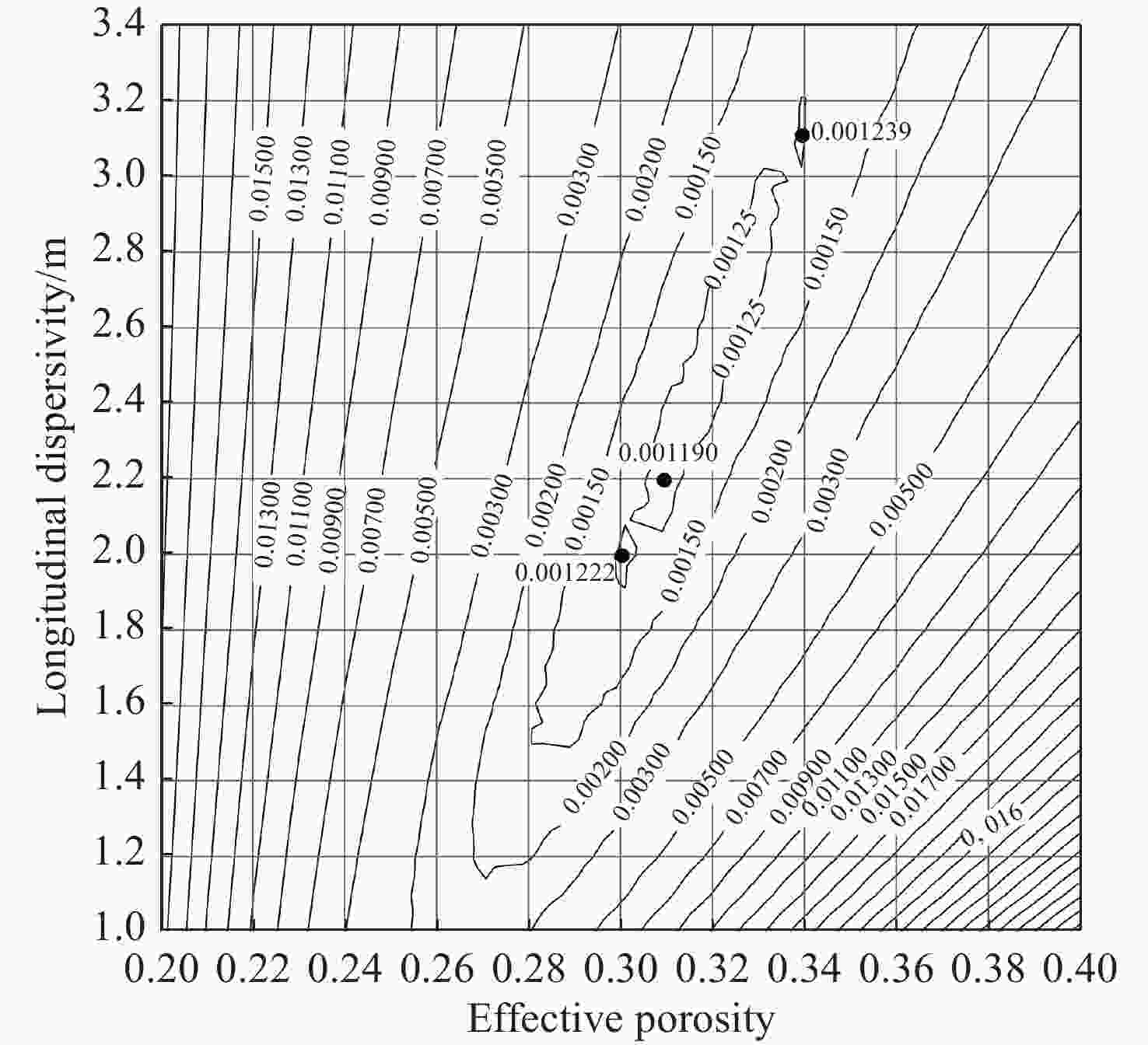
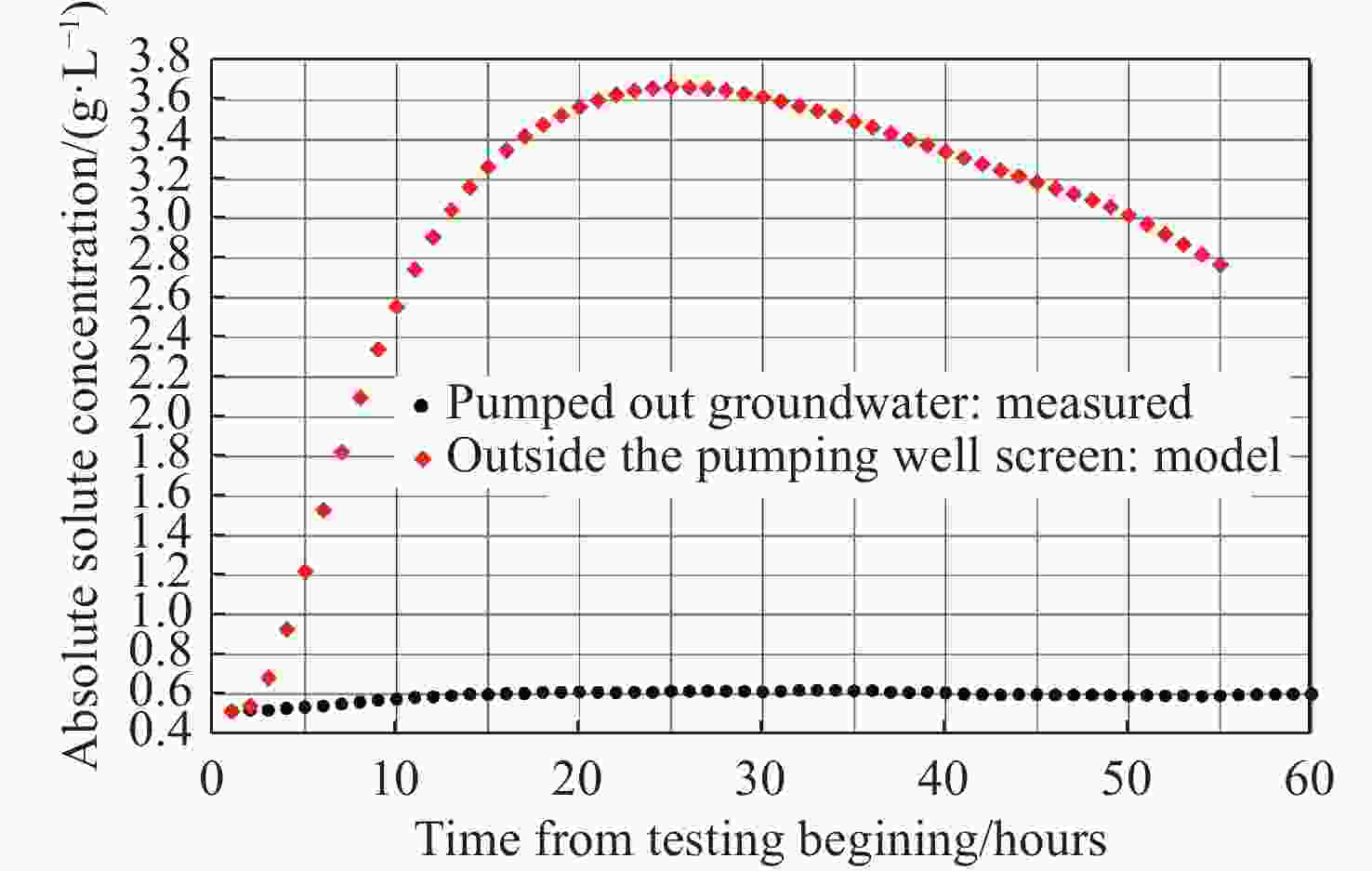
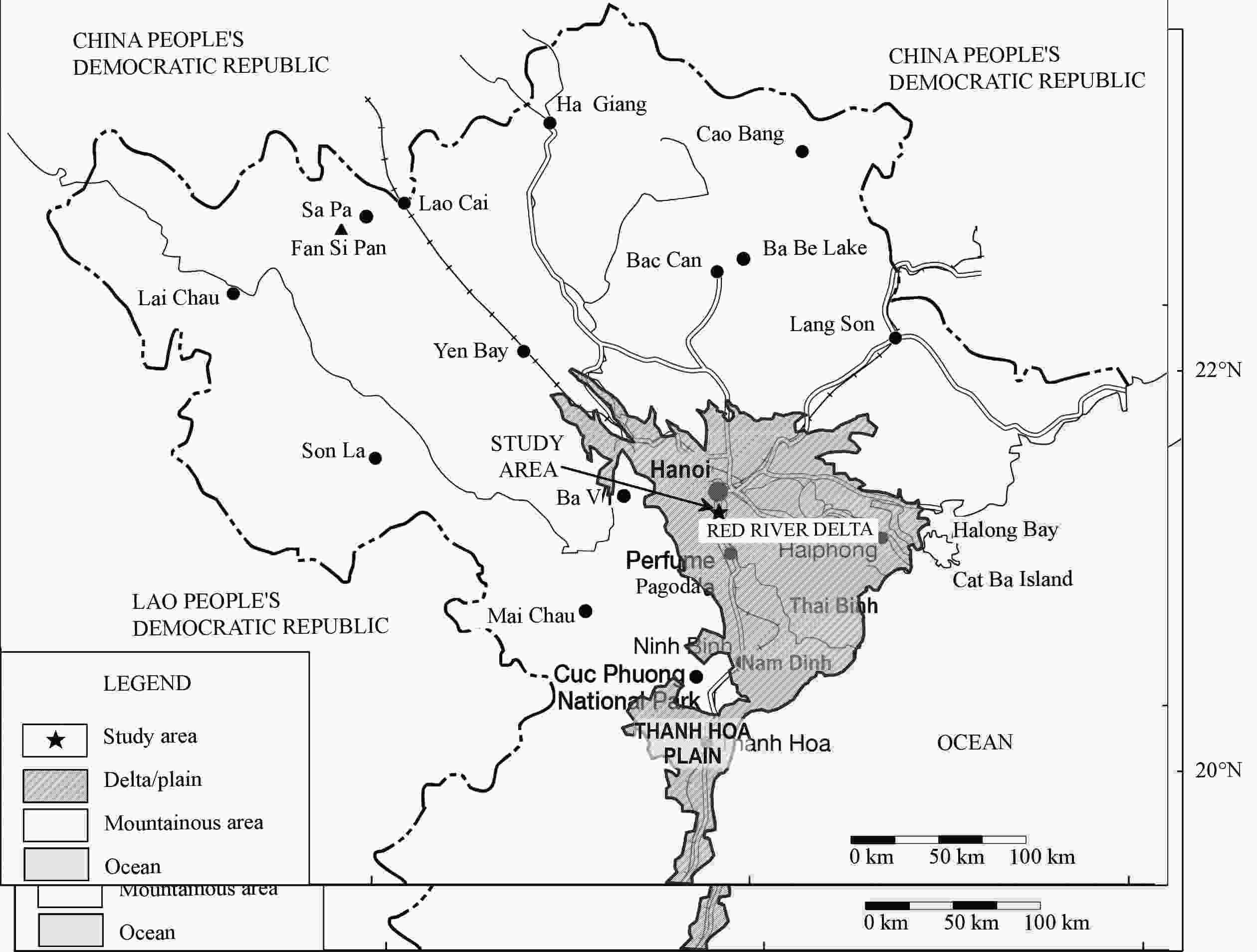
Abstract: The groundwater tracer injection and withdrawal tests are often carried out for the determination of aquifer solute transport parameters. However, the parameter analyses encounter a great difficulty due to the radial flow nature and the variability of the temporal boundary conditions. An adaptive methodology for the determination of groundwater solute transport parameters using tracer injection and withdrawal test data had been developed and illustrated through an actual case. The methodology includes the treatment of the tracer boundary condition at the tracer injection well, the normalization of tracer concentration, the groundwater solute transport finite element modelling and the method of least squares to optimize the parameters. An application of this methodology was carried out in a field test in the South of Hanoi city. The tested aquifer is Pleistocene aquifer, which is a main aquifer and has been providing domestic water supply to the city since the French time. Effective porosity of 0.31, longitudinal dispersivity of 2.2 m, and hydrodynamic dispersion coefficients from D = 220 m2/d right outside the pumping well screen to D =15.8 m2/d right outside the tracer injection well screen have been obtained for the aquifer at the test site. The minimal sum of squares of the differences between the observed and model normalized tracer concentration is 0.00119, which is corresponding to the average absolute difference between observed and model normalized concentrations of 0.035 5 (while 1 is the worst and 0 is the best fit).
-
Key words:
- Groundwater solute transport /
- Tracer injection /
- Effective porosity /
- Longitudinal dispersivity /
- Flow distortion coefficient /
- Normalized concentration
①Tong Thanh Tung. 2015. Specialized report: Interpretation and analysis of aquifer parameters for pumping test at group-well test CHN5 in Nghiem Xuyen-Thuong Tin-Hanoi. Project “Groundwater protection in large cities (city: Hanoi)”. Vietnam National Center for Water Resources Planning and Investigation-MoNRE.
注释: -
Table 1. Estimated hydraulic parameters of the Pleistocene aquifer and well information in the testing area
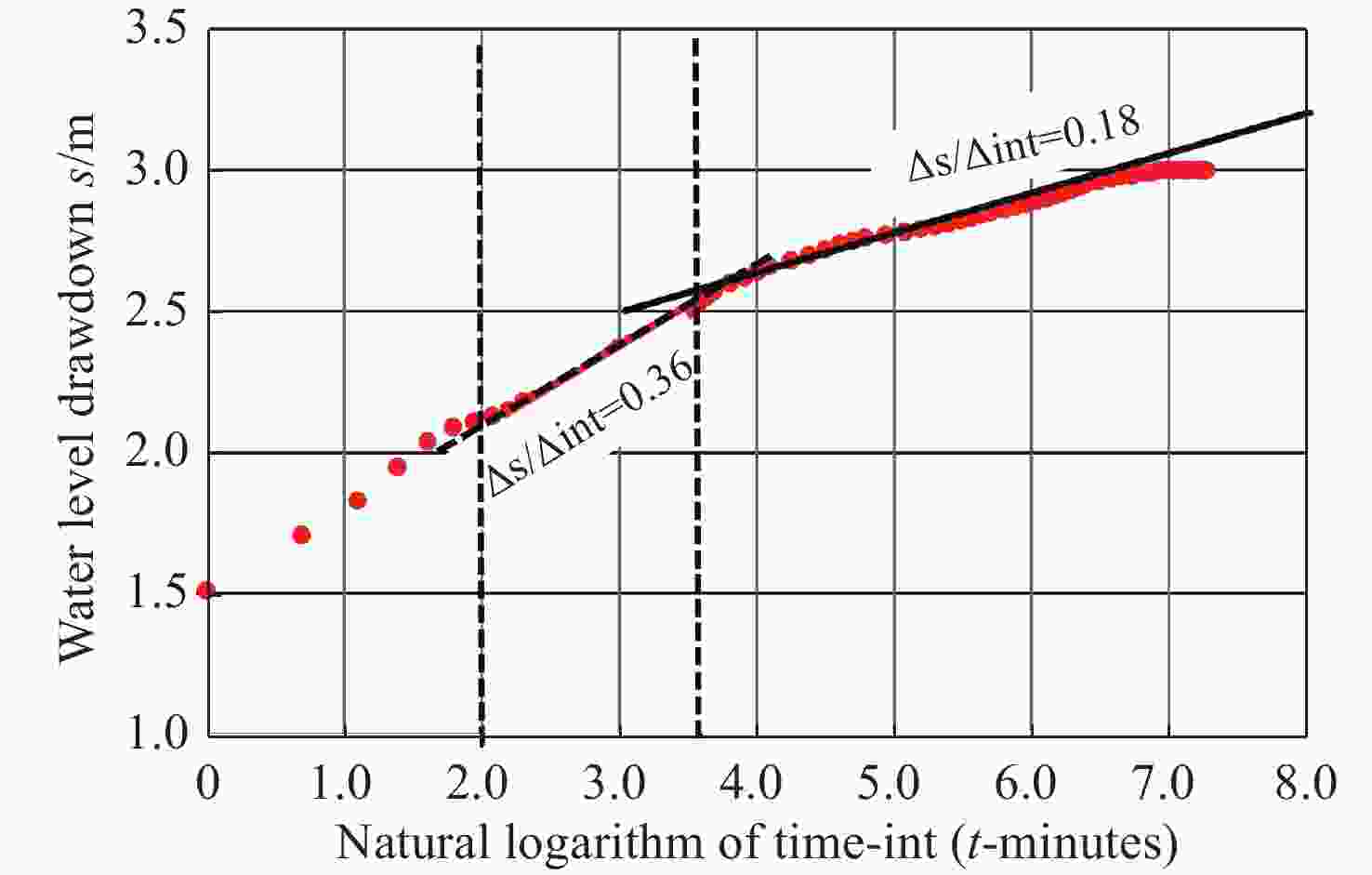
Well Aquifer Well’s capacity (L/s) Drawdown (m) Hydraulic conductivity (m/d) Transmissivity (m2/d) LK114 qh 3.33 2.54 12.19 210 LK140A qp 13.20 7.88 20.71 290 LK141 qp 12.62 1.03 70.00 1 610 LK119A qh 3.84 1.15 9.20 260 LK120 qp 11.48 0.78 152.00 1 670 LK121 qp 12.33 2.05 36.82 630 LK122 qp 6.06 15.17 12.80 80 LK104 qp 6.67 2.35 22.68 410 LK101 qp 9.09 5.04 23.14 240 LK102 qp 12.50 0.87 58.05 1 680 LK103 qh 8.33 3.47 9.70 330 LK129 qp 21.79 1.09 83.53 2 510 LK110 qp 7.82 4.04 18.28 210 LK130 qh 0.40 16.19 Table 2. The average least squares and corresponding effective porosity and longitudinal dispersivity
neff aL (m) Average least squares neff aL (m) Average least squares neff aL (m) Average least squares 0.26 1.80 0.003 728 0.29 2.70 0.002 551 0.33 2.30 0.001 569 0.26 1.90 0.004 006 0.29 2.80 0.002 734 0.33 2.40 0.001 429 0.26 2.00 0.004 286 0.29 2.90 0.002 919 0.33 2.50 0.001 329 0.26 2.10 0.004 565 0.29 3.00 0.003 104 0.33 2.60 0.001 264 0.26 2.20 0.004 840 0.30 1.80 0.001 288 0.33 2.70 0.001 227 0.26 2.30 0.005 111 0.30 1.90 0.001 231 0.33 2.80 0.001 212 0.26 2.40 0.005 377 0.30 2.00 0.001 222 0.33 2.90 0.001 217 0.26 2.50 0.005 636 0.30 2.10 0.001 253 0.33 3.00 0.001 239 0.26 2.60 0.005 889 0.30 2.20 0.001 314 0.34 1.80 0.004 463 0.26 2.70 0.006 136 0.30 2.30 0.001 399 0.34 1.90 0.003 792 0.26 2.80 0.006 375 0.30 2.40 0.001 500 0.34 2.00 0.003 239 0.26 2.90 0.006 608 0.30 2.50 0.001 615 0.34 2.10 0.002 785 0.26 3.00 0.006 832 0.30 2.60 0.001 743 0.34 2.20 0.002 414 0.27 1.80 0.002 522 0.30 2.70 0.001 880 0.34 2.30 0.002 113 0.27 1.90 0.002 746 0.30 2.80 0.002 023 0.34 2.40 0.001 871 0.27 2.00 0.002 980 0.30 2.90 0.002 172 0.34 2.50 0.001 679 0.27 2.10 0.003 220 0.30 3.00 0.002 325 0.34 2.60 0.001 530 0.27 2.20 0.003 463 0.31 1.80 0.001 608 0.34 2.70 0.001 417 0.27 2.30 0.003 706 0.31 1.90 0.001 418 0.34 2.80 0.001 336 0.27 2.40 0.003 948 0.31 2.00 0.001 293 0.34 2.90 0.001 281 0.27 2.50 0.004 188 0.31 2.10 0.001 220 0.34 3.00 0.001 250 0.27 2.60 0.004 424 0.31 2.20 0.001 190 0.34 3.10 0.001 239 0.27 2.70 0.004 656 0.31 2.30 0.001 194 0.35 1.80 0.005 981 0.27 2.80 0.004 884 0.31 2.40 0.001 227 0.35 1.90 0.005 125 0.27 2.90 0.005 107 0.31 2.50 0.001 282 0.35 2.00 0.004 407 0.27 3.00 0.005 325 0.31 2.60 0.001 357 0.35 2.10 0.003 803 0.28 1.80 0.001 720 0.31 2.70 0.001 446 0.35 2.20 0.003 298 0.28 1.90 0.001 871 0.31 2.80 0.001 549 0.35 2.30 0.002 876 0.28 2.00 0.002 043 0.31 2.90 0.001 660 0.35 2.40 0.002 525 0.28 2.10 0.002 231 0.31 3.00 0.001 774 0.35 2.50 0.002 235 0.28 2.20 0.002 430 0.32 1.80 0.002 257 0.35 2.60 0.001 997 0.28 2.30 0.002 637 0.32 1.90 0.001 922 0.35 2.70 0.001 802 0.28 2.40 0.002 849 0.32 2.00 0.001 668 0.35 2.80 0.001 645 0.28 2.50 0.003 063 0.32 2.10 0.001 482 0.35 2.90 0.001 521 0.28 2.60 0.003 274 0.32 2.20 0.001 348 0.35 3.00 0.001 426 0.28 2.70 0.003 482 0.32 2.30 0.001 260 0.36 1.80 0.007 748 0.28 2.80 0.003 689 0.32 2.40 0.001 210 0.36 1.90 0.006 698 0.28 2.90 0.003 894 0.32 2.50 0.001 191 0.36 2.00 0.005 805 0.28 3.00 0.004 096 0.32 2.60 0.001 198 0.36 2.10 0.005 044 0.29 1.80 0.001 320 0.32 2.70 0.001 226 0.36 2.20 0.004 397 0.29 1.90 0.001 375 0.32 2.80 0.001 273 0.36 2.30 0.003 846 0.29 2.00 0.001 463 0.32 2.90 0.001 335 0.36 2.40 0.003 378 0.29 2.10 0.001 578 0.32 3.00 0.001 409 0.36 2.50 0.002 980 0.29 2.20 0.001 713 0.33 1.80 0.003 215 0.36 2.60 0.002 645 0.29 2.30 0.001 863 0.33 1.90 0.002 718 0.36 2.70 0.002 363 0.29 2.40 0.002 025 0.33 2.00 0.002 320 0.36 2.80 0.002 126 0.29 2.50 0.002 195 0.33 2.10 0.002 005 0.36 2.90 0.001 931 0.29 2.60 0.002 371 0.33 2.20 0.001 758 0.36 3.00 0.001 769 -
Barone FS, Rowe RK, Quigley RM. 1992. A laboratory estimation of diffusion and adsorption coefficients for several volatile organics in natural clayey soils. Journal of Contaminant Hydrology, 10(3): 225-250. doi: 10.1016/0169-7722(92)90062-J Bear J, Verruijt A. 1987. Modeling groundwater flow and pollution. D Reidel Publishing Company, Dordrecht: Holland. Brouyère S. 2003. Modeling tracer injection and well-aquifer interactions: A new mathematical and numerical approach. Water Resource Research, 39(3): 1070-1075. doi: 10.1029/2002WR001813 Do Van Binh. 2013. Source and formation of the arsenic in ground water in Hanoi, Vietnam. Journal of Groundwater Science and Engineering, 1(1): 102-108. Dong P. 1998. Mathematical modelling of coastal processes. Proceedings of the Forty Ninth Scottish Universities “Physical processes in the Coastal Zone: Computer modelling and remote sensing”. 95-108. Drost W, Klotz D, Koch A et al. 1968. Point dilution methods of investigating ground water flow by means of radioisotopes. Water Resource Research, 4(1): 125-146. doi: 10.1029/WR004i001p00125 Fetter CW. 2001. Applied Hydrogeology. Prentice Hall Inc. New Jersey 07458. Fried JJ. 1975. Groundwater pollution: Theory, methodology, modelling, and practical rules. Elsevier Scientific Publishing Company. 330. Gelhar LW, Welty C, Rehfeldt KR. 1992. A critical review of data on field-scale dispersion in aquifers. Water Resource Research, 28(7): 1955-1975. doi: 10.1029/92WR00607 Glen BC, Welty C, Buxton HT. 1999. Design and analysis of tracer tests to determine effective porosity and dispersivity in fractured sedimentary rocks, Newark basin, New Jersey. US Geological Survey. Water-Resources Investigations Report 98-4126A. Gutierrez A, Klinka T, Thiery D, et al. 2013. TRAC, a collaborative computer tool for tracer-test interpretation. EPJ Web of Conferences 50: 03002. doi: 10.1051/epjconf/20135003002 Hall SH. 1996. Practical single-well tracer methods for aquifer testing. In: Tenth National Outdoor Action Conference and Exposition, National Groundwater Association, Columbus, Ohio, USA, 11. Honjo Y, Sato K, Nguyen Van Hoang. 1997. Groundwater of Hanoi, Vietnam: Parameter identification by the Bayesian method. Proceedings of Theme C: Groundwater: An Endangered Resource (463-468), the 27th IAHR Congress California, USA. Huyakorn PS, Pinder GF. 1983. Computational methods in subsurface flow. Academic Press, New York: 473. Keisuke K, Takeshi H, An Thuan Do, et al. 2017. Groundwater recharge in suburban areas of Hanoi, Vietnam: Effect of decreasing surface-water bodies and land-use change. Hydrogeology Journal, 25: 727-742. Klotz D, Seiler K P, Moser H. 1980. Dispersivity and velocity relationship from laboratory and field experiments. Journal of Hydrology, 45(4): 169-184. Martyna G, Emiliano S, Daniel S, et al. 2021. Arsenic behavior in groundwater in Hanoi (Vietnam) influenced by a complex biogeochemical network of iron, methane, and sulfur cycling. Journal of Hazardous Materials, 407(5). Nguyen Van Hoang. 2018. Study on the finite element modeling software for simulation of groundwater flow and solute transport by groundwater-application to aquifer in central plain of Vietnam. Codded ĐT. NCCB-ĐHƯD. 2012-G/04 supported by NAFOSTED-MOST. Novakowski KS. 1992. An evaluation of boundary conditions for one-dimensional solute transport: 1-Mathematical development. Water Resource Research, 28(9): 2399-2410. doi: 10.1029/92WR00593 Rifai MNE, Kaufman WJ, Todd DK. 1956. Dispersion phenomena in laminar flow through porous media. Sanitary Engineering Research Laboratory and Division of Civil Engineering, University of California, 93(2): 157. Sharma PK, Sawant VA, Shukla SK, et al. 2014. Experimental and numerical simulation of contaminant transport through layered soil. International Journal of Geotechnical Engineering, 8(4): 345-351. doi: 10.1179/1939787913Y.0000000014 Shook GM, Ansley SL, Wylie A. 2004. Tracers and Tracer Testing: Design, Implementation, and Interpretation Methods. US Department of Energy Office of Environmental Management. Report NEEL/EXT-03-01466. Welty C, Gelhar LW. 1994. Evaluation of longitudinal dispersivity from non-uniform flow tracer tests. Journal of Hydrology, 153(1): 71-102. Yan X, Qian J, Ma L. 2019. Experimental study on the velocity-dependent dispersion of the solute transport in different porous media. Journal of Groundwater Science and Engineering, 7(2): 106-114. Zhou L. 2002. Solute transport in layered and heterogeneous soils. Louisiana State University and Agricultural and Mechanical College. Ph. D. Thesis. Zhu H, Jia C, Xu Y, et al. 2018. Study on numerical simulation of organic pollutant transport in groundwater northwest of Laixi. Journal of Groundwater Science and Engineering, 6(4): 106-1114. Zienkiewicz OC, Morgan K. 1983. Finite Elements and Approximation. Academic Press. Zlotnik VA, Logan J D. 1996. Boundary Conditions for Convergent Radial Tracer Tests and Effect of Well Bore Mixing Volume. Papers in the Earth and Atmospheric Sciences: 159. -
 E-mail alert
E-mail alert Rss
Rss


 下载:
下载: